Exploring the Two Possible Solutions in Algebra Puzzles
Written on
Chapter 1: The Challenge of Finding Solutions
At first glance, this may seem like a straightforward algebraic conundrum. However, upon deeper analysis, you may uncover that there are actually two distinct solutions! The pivotal question remains: what are those solutions?
Before proceeding, I encourage you to take a moment, grab your pen and paper, and attempt to solve this puzzle on your own. Once you feel prepared, continue reading for the resolution!
Solution Approach
Initially, I believed that substituting ( x = 2 ) into the equation would suffice, as it leads to ( 2^2 - 2 = 4 - 2 = 2 ), which gives us ( frac{3}{2} ). Yet, this is only part of the answer!
A more comprehensive method involves recognizing the quadratic form of the equation ( x^2 - x ). Our goal is to set it equal to 2, as demonstrated below.
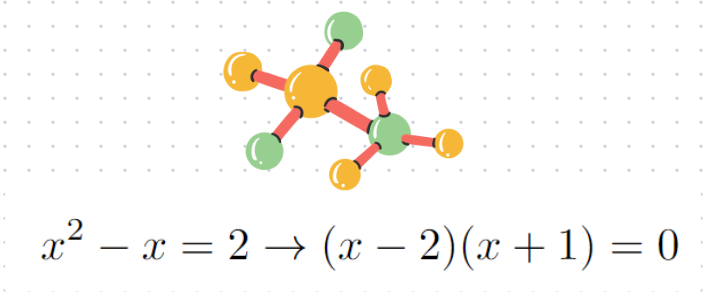
This indicates that there are two specific values for ( x ) that satisfy the equation ( x^2 - x = 2 ). Those values are ( 2 ) and ( -1 ). Thus, we conclude that there are indeed two solutions.

And that leads us to our final answer.

Photo by Vincentiu Solomon on Unsplash
How fascinating is that?
What thoughts crossed your mind during this process? I would love to hear your insights in the comments below!
Math Puzzles
Explore an array of engaging math puzzles on Medium, spanning various topics including Algebra, Geometry, Calculus, and Number Theory.
Bella’s Weekly Math Games
Join in on a 48-hour math competition every week!
Stay Updated
Sign up to receive notifications whenever I publish new content. Thank you for your support!
Thank you for taking the time to read this article. If you found it valuable, please give it a clap!
If you feel inclined, I would greatly appreciate your support! I invest considerable effort into each article, and a small gesture like buying me a coffee would mean the world. Your generosity helps sustain my writing, personal, and academic endeavors.
Happy Solving, Bella 😊